Why Fmin in cascode LNA doesn't affect by the feedback inductor?
Actually the noise factor IS affected by the degeneration source inductor in a cascode LNA.
I am reading this paper and in formula (11 ) page 3, it shows that minimum noise factor Fmin isn't affected by Ls. Please look at this:
http://nice.kaist.ac.kr:8080/pdf/journals/2004/1.pdf
Assuming the input and output noise of the transistor are correlated, and the inductor is lossless, then I don't see how the inductor could possibly change Fmin. But it will change how Fmin is actually achieved.
In Eqs. (9)-(11), the noise parameters with superscripted
zeros are those of the cascode amplifier with no degeneration
(see Eqs. (5)-(7))
You confuse Fmin and F. Fmin is of course independent from Ls because it's intrinsic parameter of the transistor and depends on model parameters only.But F is dependent on those parameters and source degeneration inductor.Thus you approach to Fmin by implementing a proper inductor value.
Thanks, I know the difference between F and Fmin. What confused me is why Fmin is independent on Ls. I tried a lot to calculate Fmin as in the file but didn't get that result. In my calculation
Fmin depends on Ls. Fmin increases if Ls increases.
At the APPENDIX they gave a proof for that formula. I followed it and proved formulas from A1 - A4. However, I can't get the formula in A9.
Could you tell me how to calculate this in A7?
|Io, ngc + Io, nd|^2
In A9, I know that there is a mistake there in (Rs)^2 - s(Ls)^2. It should be (Rs)^2 - (sLs)^2. I want to derive that formula myself to get a good understanding.
Hello,
Ls is lossless; it doesn't contribute noise source to the circuit but it changes the circuit topology and therefore changes voltage gain and finally changes Fmin. That is my thought.
By the way, is there a way to find Fmin of a circuit without knowing F? As BigBoss said Fmin doesn't depend on Ls because it's intrinsic parameter of the transistor and depends on model parameters only, I wonder why Fmin has such a meaning? I have searched a lot but can't find that definition.
Hello,
Ls is lossless; it doesn't contribute noise source to the circuit but it changes the circuit topology and therefore changes voltage gain and finally changes Fmin. That is my thought.
By the way, is there a way to find Fmin of a circuit without knowing F? As BigBoss said Fmin doesn't depend on Ls because it's intrinsic parameter of the transistor and depends on model parameters only, I wonder why Fmin has such a meaning? I have searched a lot but can't find that definition.
Why would changing the gain necessarily change Fmin?
Because it's a very useful metric for selecting LNA transistors.
Because when we add Ls into the circuit, the circuit is no longer a cascode but a cascode degradation. Therefore, its gain also changes. The added Ls doesn't change noise source because it is losses. As gain changes, the input-referred noise (current and voltage sources) also changes => F changes.
I tried to find F expression and then from that find Fmin. I see that Fmin depends on Ls.
May I know where to find that definition?
Why would a change in gain necessarily change input referred noise? For example, if I take a matched LNA and measure its NF, then add an attenuator on the output and measure it again, I get the same NF, right? Of course, adding a feedback component is not the same thing, but that has nothing to do with the gain in the overall amplifier.
As far as I understand it, Fmin is always derived from source pull analysis (simulated or experimental). You sweep your source impedance and measure F over the entire smith chart, and the minimum value from that F dataset is Fmin.
To be clear, I'm not convinced that you're wrong, but I don't find your reasoning convincing.
It would be great if you could help me with this. I write this post based on the book "The Design of CMOS Radio-Frequency Integrated Circuits" by Thomas H. Lee.
Here is a summary about noise factor of a two-port network.
A noisy two-port network is represented as follows.
And here is the equivalent noise model. All of the noise appears as inputs to the noiseless network.
The noise factor:
And its optimum value Fmin:
And F is also expressed in terms of Fmin as follows:
Now I want to apply the formula for my cascode LNA circuit. And in order to use that formula I need to find input-referred noise sources ("en" and "in" as in the picture).

The first step: convert channel thermal noise "id" from output to input. The current noise is converted to input by the voltage source "en".
As we add Ls into the circuit, the gain of overall circuit changes => "en" also changes.
"en" changes => F changes.
From "en" and "in" we can easily write F and Fmin functions.
I did all calculations above but didn't get Fmin correct (as in the paper said that Fmin doesn't depend on Ls but in my final formula, it does depend. That is where I am confused.
Hope you can point out where I am wrong. I have got stuck at this for more than two weeks and really don't know why it is wrong, why Fmin doesn't depend on Ls. If you know any proof about that, please let me know.
I attached the pictures about my calculation here. Hope experts can help me out.


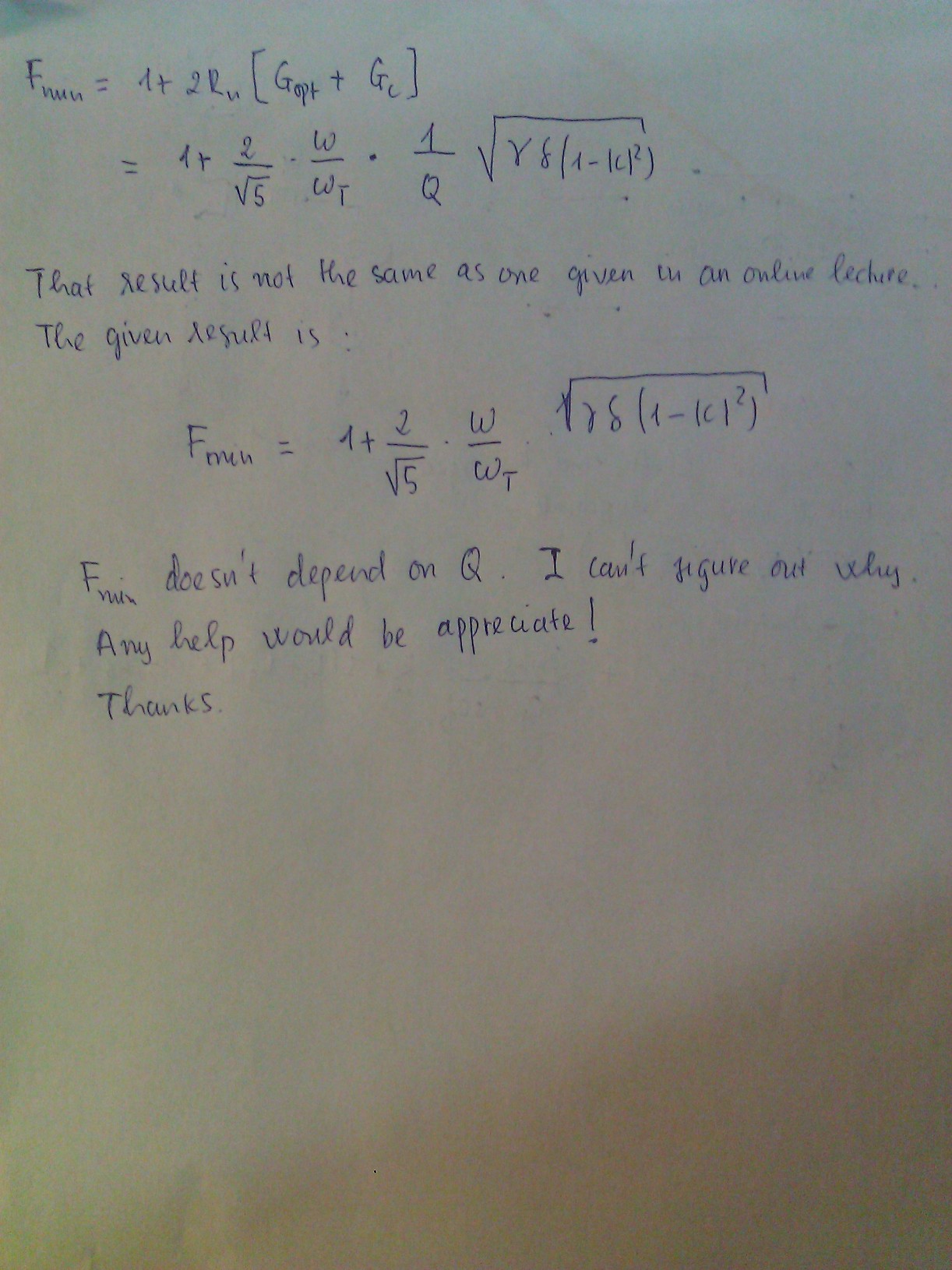
I guess my network analysis is rusty, I'm not familiar with most of those symbols. I assume Ys is source admittance and Yc is Y11 of the network? What are iu, Gc, Bs, Bc, etc?
